From the Bridges of Königsberg – Why Topology Matters in Psychoanalysis
This is the first of two articles that will explore Lacan’s idea that human subjectivity has the structure of a topological space.
In the early eighteenth century the city of Königsberg, now part of modern-day Russia, was connected by seven bridges which linked the two islands of the city with each other and the mainland.
The citizens of Königsberg had posed themselves the following problem: was there a way to walk through the city by crossing each bridge fully once and only once?
In 1735 a young Swiss mathematician, Leonhard Euler, decided to have a go.
His idea was that the map of Königsberg could be stripped of all its geographical attributes and isolated to just a few key features: the land masses which made up the city (vertices or nodes) and the connection points between them, the bridges themselves (edges). What you get is the distillation of a geography into a mathematical structure called a graph:
If all you’re interested in are the nodes and edges of this graph, you can distort, bend, transform, and reconfigure the city as much as you like and it will still retains these essential properties. For Euler, it was not the position of the elements on the map of Königsberg that mattered but rather simply the number of nodes and edges.
With this distillation, Euler solved the problem and showed that it couldn’t be done: it was not possible to walk the city by crossing each bridge fully once and once only. The methodology that he used to prove this evolved into what became the branch of mathematics known as topology.
Fast forward almost two hundred years and Freud had a similar problem when he came to describe the workings of the human psyche. Much like the map of Königsberg, Freud was trying to represent what he referred to as “psychical locality” (SE V, 536). However unlike the terrain of a city, Freud was very careful to point out that by ‘psychical localities’ he was not referring to areas in the brain, but rather using topographical schemas.
To represent this Freud developed two models of the human psyche over the course of his life: the ‘first topography’ (conscious/preconscious/unconscious) and the ‘second topography’ (id/ego/superego):
But Freud was never satisfied with these, referring to them as his ‘witch metapsychology’ (SE XXIII, 225). It was always, for him, an imperfect representation of the realities of psychical life he believed he had discovered with psychoanalysis.
Lacan however, picked up this challenge. As we will see, he worked throughout his life with various topological forms, but it was towards the end of his life that his interest in topology intensified. Throughout the 1970s he busied himself with rings of string, weaving knots and lattices in an attempt to construct a topological model that represented the human soul. Referring to one topological form which especially fascinated him during these years – the Borromean knot – his biographer Elisabeth Roudinesco wrote that these were the years that Lacan lived on “planet Borromeo” (p.377).
Lacan surrounded himself with a small group of fiercely intelligent young mathematicians to conduct this work. Michel Thomé and Jean-Michel Vappereau both exchanged around two hundred letters with Lacan on the subject of topology, with Vappereau being invited by Lacan to give a lecture on the Borromean knot at his seminar in 1978.
But first among these collaborators was Pierre Soury, who Lacan credits in Seminar XXIV with providing the help he needed to pursue his topological adventures. Throughout the mid-seventies the pair also exchanged a huge volume of letters featuring diagrams of topological models, like a four-ring Borromean knot, that they were trying to construct:
Tragically, Soury killed himself in July 1981 having never been able to start an analysis with Lacan, despite his repeated requests. Before drinking three bottles of cyanide concoction, he left a suicide note that read ‘I am tired of living alone’. Lacan himself died a few weeks later.
Briefly, we can give three reasons why topology so fascinated Lacan in his later years:
1. Topology offers a way of thinking about any particular space whatsoever. The human reality of our everyday lives can be considered as a topology, composed of points, arranged into neighbourhoods, within sets. In the second part of this series we will look more closely at how, but for now we can simply say that any kind of differential space – geographical space, signifying space, any reality you come across – can be conceptualised in this form. Take the space constituted by the chain of signifiers that most students of Lacan’s work are familiar with. As one of his topological collaborators Pierre Skriabine notes, the key thing about a signifying network is difference – a signifying matrix can be built only if one signifier is distinguished from another. It doesn’t matter what the signifier actually is (a word, a marking, a number, or a piece on a chessboard) all that matters is that it is different from the others; that there is a space of some sort between them, so that this space can be considered in terms of the differential properties which compose it (p.81).
2. Therefore, as Freud had sensed, psychic and material space cannot be separated. And topology offered Lacan a way of showing how they don’t have to be. Freud’s problem, as we saw above, was that he was trying to represent the unconscious – topographically, structurally, dynamically – solely with recourse to models based on the purely two-dimensional and three-dimensional spaces of Euclidean geometry.
3. However, just as we saw with the seven bridges of Königsberg, the hallmark of topology is that it deals with figures which retain their properties regardless of their deformations. You can bend and stretch and manipulate topological space as much as you like but it still retains its essential topologically significant characteristics. A Klein bottle, for instance, like a Mobius band, is a non-orientable surface – you can reach into it and pull the inside to the outside (see for example this little video for some of the many things you can do with a Klein bottle). To take another example, in Seminar XXIV Lacan opens one session by telling his audience he has been playing with tresses of string for the past 48 hours to make different configurations of the four-ringed Borromean knot which represents how his Real, Imaginary and Symbolic registers are held together (Seminar XXIV, 18th January 1977).
Here are some he made:
Let’s look at these three points in more detail using a couple of the topological models Lacan was so interested in.
Two references to Rome – one from Freud and one from Lacan.
Freud was a great student of ancient Rome and longed to go there himself (something his hero Hannibal was never able to do). At the time of writing Civilisation and its Discontents he was reading Oxford scholar Hugh Last’s essay ‘The Founding of Ancient Rome’, and Freud describes in his paper the successive iterations of the Eternal City, from a fenced settlement on the Palatine, to a city bound by the Servian and then Aurelian walls (SE XXI, 69). In Rome there are ruins, and there are ruins of the restorations of the ruins.
Having earlier proposed the idea that the unconscious knows no time, no death, no negation, Freud asks the reader to imagine – what if Rome was like our unconscious? We would find everything that has happened in its history all there at once, effectively on the same level. Time and space would not matter, everything would be folded on top of one another. But then Freud acknowledges how absurd this would be:
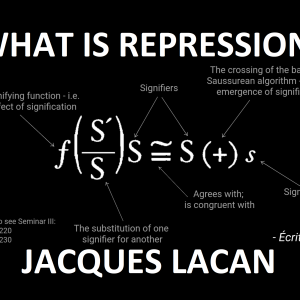
We see why Freud needed a topological representation so badly. Here he clearly recognises the uselessness of temporal or spatial terms to describe the unconscious. Look, for instance, at the problem that Freud had with a concept like repression. Why is something repressed in the first place? In 1915, the hypothesis of primary repression is Freud’s answer. There must be something primally repressed that exerts an anticathexis to keep what is repressed repressed, and to draw towards it other material (SE XIV, 181). Indeed, all of the so-called Ur- phenomena (primal repression, primal father, primal phantasy) that Freud relies on to support his metapsychology are employed to re-present the problem of why the unconscious does not seem to observe the rules of linear time and space (before/after, here/there, etc). A topological model, on the other hand, would have enabled Freud to conceive of representations, memory traces, psychical impressions, in another way.
Lacan went to Rome himself in 1953 to deliver his manifesto for psychoanalysis – the Rome Discourse, cornerstone of the so-called ‘Return to Freud’. It contained his first reference to the use of topology in psychoanalysis (Écrits, 320-321) via a topological model he kept coming back to again and again: the torus.
By the time he returned to Rome in 1974 he was deep into the topological models that would define his late teaching.
If we take one representation he gives of the torus from around this time – Seminar XXIV in 1976 – we can see how it provides an alternative solution to the problems of psychical structure and human subjectivity which so beset Freud:
Drawing the image above on the blackboard at his seminar, Lacan showed his audience how the torus and the Mobius band are continuous – they have the same topological properties. The torus can be cut, and when it is cut can become a double Mobius strip, with one inside the other.
How does this help us solve some of Freud’s problems?
If we look at the Mobius band, for instance, we see that both the inside and the outside are continuous. If you traced your finger around the surface of the Mobius band you would move from the top to the bottom seamlessly, without lifting your finger. Or without lifting your ant, as the cover of Seminar X illustrates:
Lacan’s idea is that this is what the unconscious looks like. We don’t have a divide between an ‘above’ and ‘below’, or between a surface and a depth. The unconscious is not hidden in the deep and the surface is not the superficial. It is, to use Lacan’s clever expression, ‘extimate’, both external and intimate at the same time. Describing the Mobius band in Seminar XXIV he says, “It is very precisely what is going to give us an image of what is involved in the link between the conscious and the unconscious.” (Seminar XXIV, 14th December 1976).
We find the same thing with the torus. In the Rome Discourse, Lacan describes how “a torus’ peripheral exteriority and central exteriority constitute but one single region.” (Écrits, 321). If you make a cut in a torus, you can pull the inside to the outside to produce an ‘inverted’ torus, but which still has the same properties. For Lacan’s son-in-law Jacques-Alain Miller, “The torus is introduced as a figure that allows the fundamental relationship of internal exclusion to be sustained” (p.31).
We can use the model of the torus or the Mobius band to think about psychoanalytical ideas other than the unconscious, too. Take for example Lacan’s idea that the ego is constituted through an alienating identification with the other, our semblable. Here again we see that the distinction between self and other dissolves when thought of like the surface of a Mobius band. Phenomena as seemingly diverse as paranoiac persecution and love share this quality, and are thus closer to each other clinically than they might at first appear.
Or the phenomena of unconscious inter-generational transmission that Lacan saw at the heart of the Rat Man’s neurosis. A family drama from another generation appears to be ciphered and re-transcribed into the present day of a subject’s neurotic suffering. When Lacan looked at the Rat Man’s case in the early 1950s, his comparison was to the way that Levi-Strauss described the construction of a myth. But by the late 1970s, rather than turning to structural anthropology for an explanation, Lacan is thinking about these clinical examples topologically. He even says in Seminar XXIV that all of Freud’s female hysterics’ relations to their fathers can be represented topologically, as a line through a torus (Seminar XXIV, 14th December 1976).
Neurosis itself is toric for the Lacan of the late seventies. In his astonishingly difficult and dense late text L’Étourdit Lacan talks about “the neurotic torus”:
Human suffering itself exhibits the characteristics of a toric loop: “Man goes round in circles because the structure, the structure of man, is toric”, Lacan says in 1976, before adding yet another extension to his proposition: “The world is toric” (Seminar XXIV, 14th December 1976).
By quoting from his work of the early 1950s through to the late 1970s it should be clear that Lacan’s adventures in topology were not restricted to his later years of failing health, and what some believe was the onset of dementia. Topology rather provided him with an alternative approach to the same problems which, as we have seen, had a rich pedigree stretching back to Freud.
For Miller, Lacan’s intensified interest in topology in his late work can be read as part of a series alongside his other approaches which used signifiers, mathemes, schemas, and a playfulness of orthography. They all share one common quality, for Miller: the combinative. “Topology”, Miller writes, “consists of a series of matrices, of signifying combinatives” (p.35).
Coming full circle, we can see this same combinative is at stake in the bridges of Königsberg that we started with.
A signifying network, likewise, is an organised topology of combinatives. For signifiers and language we can substitute points and neighbourhoods of a topological set, and we will still be talking about the same thing. The Other is the space of combinatives that make up a topology. As for the subject, according to Miller, this remains the insubstantial subject-as-effect-of-the-signifier that we are familiar with from Lacan’s earlier work of the fifties and sixties; the only substance in psychoanalysis is jouissance:
In the second part of this series we will try to bridge the gap between all this theory and its litmus test in practical application.
We will look at how these topological theories can be applied to the real world, using clinical examples from analysts who think about their cases in these ways.
As Miller points out above, a progression from one topology to another can be used to delimit space, and it is in recognising and working with this fact that some practitioners of the Lacanian orientation have found a way of intervening therapeutically to deal with the true enemy in a psychoanalysis: jouissance.
By Owen Hewitson, LacanOnline.com

All content on LacanOnline.com is licensed under a Creative Commons Attribution-NonCommercial 3.0 Unported License.











ecuador existe porque la mision academica asi nos nomino la topologia hubiese facilitado un poco las cosas si los cartografos no la hubiesemos tenido que complicarnos con descartes y su plano base de los ejes de coordenadas y cuadriculas cuando vappereau y lew pisaron ecuador vieron que el unico sabio no era un antecesor mio don pedro vicente maldonado sino que en riobamba tambien exsten analistas estar en en esta latitud y haber tenido a los incas nos da otra concepcion de espacio y tiempo que la clasica occidental los nudos llamados kypus eran nustra matematicas. lo vieron asi mellman y czermack isignificante ecuador ombligo y petit a del mundo tres regiones continentales una insular rios y montanas forma de aprender topologia no a lo ortodoxo si a lo viviente si del psicoanalisis algo es trasmisible es lo recorrorido y lo vivido hciendo camino sal andarsolo errando se aprende y topesando con los pies lacan decia que se orientaba con sus pies pues algo queda olvidado tras de l que se dice esn esto que ustedes endienten sorry por no ser ortodoxo yo no repito lacan lo invento yo hago talleres no soy burocrata dibujar y disenar al fin al cabo carroll le wis algo me esneno si no no hubiese leido los ecritos de lacan ni mehubiese atevido a desnrdar nudos no orientables
Dear All,
Truly excellent article indeed, however, may I suggest you provide a direct link to the second article in this series, somewhere in the body of the document?
Thanks in advance and best regards.
https://www.lacanonline.com/index/2015/03/why-topology-matters-in-psychoanalysis-part-ii/
Thanks Nicolás, good point, I’ve updated the article with a link to Part II at the top. Owen
que de la suplencia del cuatro que no permite salir del coincage, al no permitir hacer un triskel mas consistente pienso que el nudo 9.40 de la tabla de rolfsen lo pudiera solucionar pero no trabajando con cuatro sino a traves de un hexadico soury diseño algo pero quedaron solo sus bocetos .bousserioux trabaja el el borro proyectivo y tambien lew, y vapperau atraves no de lo metaforico. sino mas mas bien de lo metonomico reducir la forclusion por la significancia para restablecer la intension/ extensiones.sortie de la psychoses
The braid for the brunnian link with four components is not correct. The correct braid is showed at https://math.stackexchange.com/questions/2594712/seifert-matrices-and-seifert-surfaces-for-the-following-two-brunnian-links?noredirect=1&lq=1
no es eso, que sino del triskel, que compactifica lo vacio de lo irrepresentable de la representacion no dicha de la significancia in/orientable de lo asferico del plano proyectivo+ disco el real,lo simbolico , el imaginario no son todo del sinthome de la escritura del caracter que se desrinza haciendo boucle cuatridimensional te pidos que rechazes lo que te ofresco porque no es eso.
Treinta anos despues de Soury, Leger y Thome. Para poder trabajar el borro proyectivo con Marc Saint Paul y Pierre Roth asfericidad y esfericidad predicativa e impredicativa la iconicidad incontorneable, los templetes las cadenas la cuarta dimension improyectiva e imrepresentable en 3 d mandifold links pero ni ellos creyeron que sus trabajos ivan a ser trabajados por japoneses rusos, australianos griegos y latino americanos . despues de ellos lo imposible es lo real mas alla de escher y sus dibujos
Your work is as new today and as illuminating as when you write it!!!
Ellie Ragland
raglande@missouri.edu
wrote it/sorry
Ellie